Available Research Projects 2021
Thesis topics are divided up into main research categories below. Within each category there are suggested PhD thesis research programs.
For shorter Honours and M.Sc. programs, please contact the supervisors listed below in your area of interest.
Please note that PhD scholarships are available for both international and domestic students. International prospective PhD students can also find detailed application instructions.
The next scholarship deadline is to be determined. Late applications may be considered for outstanding candidates. You will need to choose a topic and discuss this with the associated supervisor before applying.Topics that we offer fall into the following categories:
Entanglement, Nonlocality and Simulations in Quantum Mechanics
Research into these foundations of quantum theory has a wide importance both in fundamental science and also in quantum information. Quantum optics and atom-optics are uniquely placed to investigate these issues.
(1) Quantum entanglement in planar nano-mechanical systems (Margaret Reid)Nano-mechanics is an exciting opportunity in quantum physics, with macroscopic mechanical oscillators being cooled to the quantum ground state. Fist principles simulations of nanomechanical entanglement have been carried out and verified experimentally. This project will extend these simulations to study a new system: an extended array of nano-mechanical oscillators coupled to a planar cavity. Essentially, the physics involved is that of quantum acoustics. It is closely related to the science of gravity-wave detection. Here reduction of quantum noise in an extended mechanical system, namely the detection mirror, is essential to the further improvement of this important observational method. | 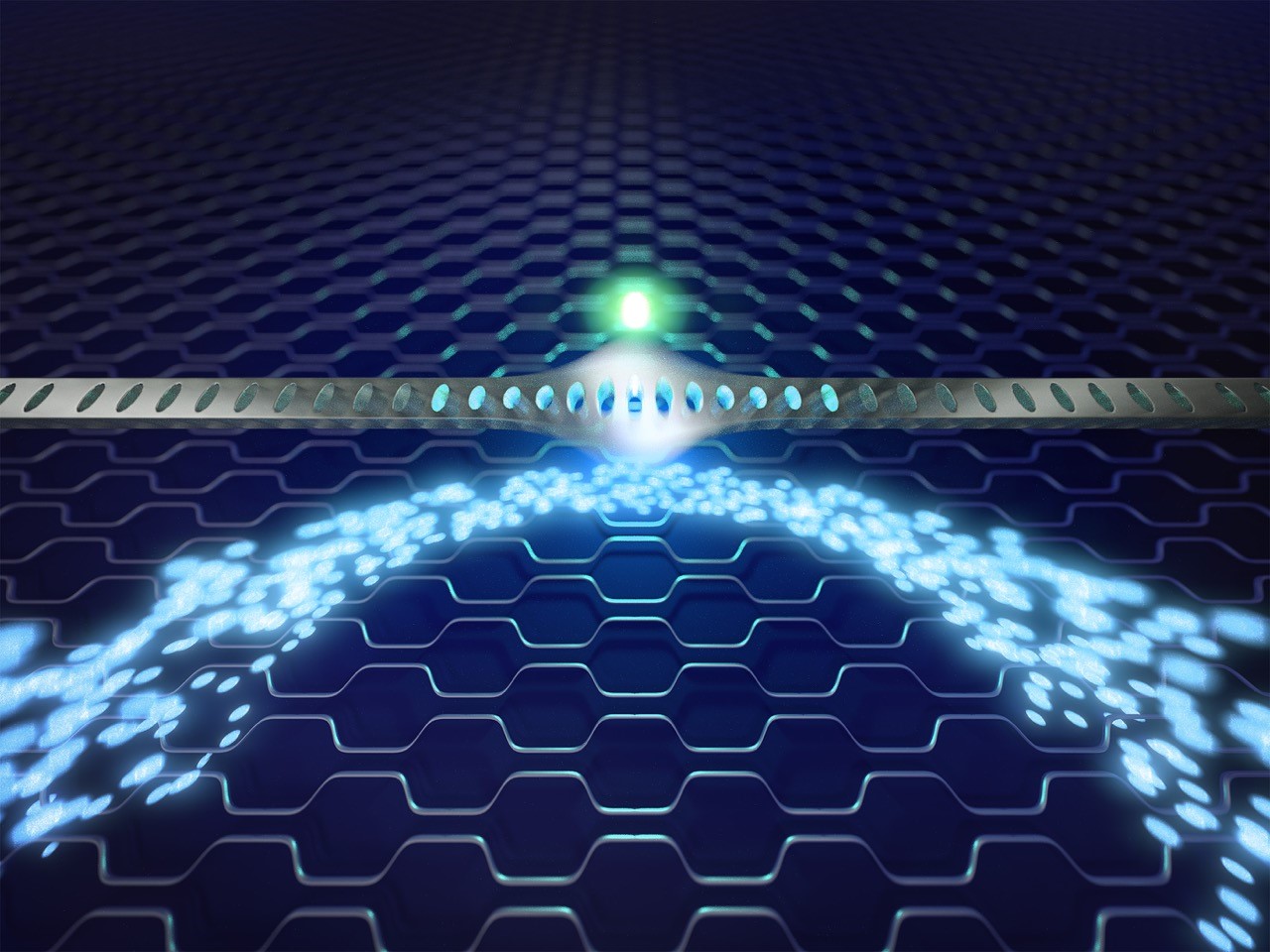 |
(2) Stochastic bridges and reversible stochastic processes (Peter Drummond)
The most challenging problem in quantum theory is the treatment of reversible, unitary evolution, in large interacting systems. The new methodology to be investigated here is the stochastic bridge: a stochastic process with defined end-points in the past and in the future. This allows time to be treated in a reversible way, thus creating a new conceptual approach to quantum dynamics. This also involves the theory of forward-backwards stochastic equations. These are a type of stochastic equation in which a diffusion process runs simultaneously forwards and backwards in time. As well as being found in financial modeling, and stochastic control theory, these equations occur in quantum systems in phase-space. The project will implement different techniques for solving these equations, and test their accuracy.
(3) Speeding up xSPDE: GPU stochastic implementations (Peter Drummond)
This project is to design and implement efficient GPU integration modules for xSPDE, a public domain stochastic integration program that integrates a range of stochastic partial differential equations. The project will evaluate the best high-level language to use, comparing Julia and Matlab, and also compare different GPU implementations. The focus is to develop a high-level implementation that is compatible with existing xSPDE inputs, while improving efficiency and speed, by a factor of 5-10 if this is feasible. Achieving this goal will require algorithm, language, and hardware changes. Algorithm improvements will include the use of spectral FFT-based methods for implementing a variety of Neuman and Dirichlet boundary conditions, as well as mixed, nonlinear and implicit boundaries in an arbitrary number of dimensions. The results will be made into public domain downloadable modules.
(4) Optimizing Ising machine hardware through simulations (Peter Drummond)
The Ising machine is a novel type of large-scale quantum computer, which solves NP-hard problems with large potential impact in many practical applications. Quantum simulations of Ising machine operation will be carried out in a joint program with the new NTT Corporation Phi laboratory in San Jose, together with Stanford. This project will be to work with a team to optimize hardware protocols. This will require developing code to simulate the quantum computer, either using phase-space methods based on stochastic equations, or using more advanced algorithms. The target hardware will include both the fiber-optical hardware currently in NTT and Stanford, as well as a new generation of superconducting quantum circuit experiments. This will involve group meetings, both by video and in person, with the Phi network researchers in USA and Japan.
(5) Quantum limits of ultra-cold plasma dynamics (Peter Drummond)
What is the quantum behaviour of an ultra-cold fermionic plasma? This project will investigate fermionic plasma dynamics in the quantum regime. The issues are to understand the coupling between the plasma components of different masses, to calculate in which regimes screening occurs, and how quantum fluctuations change this. This will involve development of novel fermionic phase-space theory to represent the quantum plasma system. The mathematical techniques are completely novel, requiring an understanding of Lie group theory, Cartan homogeneous spaces and reversible Fokker-Planck equations. The main challenge is to decompose the diffusion matrix generated by the interactions into positive and negative submatrices for path integration.
(6) Quantum paradoxes in a reality model (Margaret Reid)
Although there are many proposals for ontological models of reality underlying quantum mechanics, all previous approaches are either nonlocal or not physically viable. This project will analyse a novel proposal for a model of quantum reality and quantum measurement, that is based on phase-space representations and retro-causal trajectories in space-time. The goal of the thesis is to analyse multiple quantum paradoxes including: EPR paradoxes, Bell violations, Wigner's friend, delayed choice, contextuality, and macroscopic Leggett-Garg paradoxes. The purpose here is to demonstrate that realistic "single-universe" interpretations are possible in all these cases. This will involve constructing a measurement model in each case, then demonstrating the paradox through realistic trajectories, with either analytic or numerical dynamical solutions.
(7) Tests of macroscopic local realism and Furry’s hypothesis (Margaret Reid)
The Einstein Podolsky Rosen (EPR) paradox showed the fundamental incompatibility of the completeness of quantum mechanics with local realism. Failure of local realism may thought of as a “spooky action at a distance”. Though work by Bell later challenged the validity of local realism, this was at a microscopic level only. Local realism is thought to be the basis for theories describing macroscopic systems. Furry proposed as a possible resolution to the EPR paradox that there are inherent in nature mechanisms that mean entanglement decays with distance. The aim of this theoretical project is to quantify Einstein, Podolsky Rosen’s “action at a distance”, so that one can develop tests of mesoscopic and macroscopic, as opposed to microscopic, local realism. Hence, while Furry’s hypothesis appears wrong for microscopic systems, it can be tested at a more macroscopic level.
(8) Quantum entanglement and Bell non-locality in macroscopic systems (Bryan Dalton)
This project explores two of the strangest non-classical features of quantum physics in the least expected situation of macroscopic systems. It is relevant to the foundations of quantum theory and to technological applications in cyber security. Macroscopic Bell non-locality is being studied for double well BEC systems with two hyperfine components based on the Collins-Gisin-Linden-Massar-Popescu Bell inequalities.
Theory of Quantum Fluids
Quantum fluids appear in a diverse field of physics and can host novel exotic states of matter that may universally exist in cold atom laboratories (i.e., strongly interacting Fermi gases), new functional materials (i.e., 2D layered high-Tc superconductors and exciton- polariton condensates), and mysterious cosmic objects such as neutron stars. The understanding of these exotic states - being a long-sought theoretical challenge - is the subject of our PhD topics.
(1) Ultracold Atoms with Synthetic Spin-Orbit Coupling (Xia-Ji Liu)Recent realization of synthetic gauge fields in ultracold atoms, i.e., the creation of a spin- orbit coupling between the spin and the orbital degrees of freedom, has led to a new frontier that is endowed with a strong interdisciplinary character and a close connection to other research fields, including condensed matter physics, quantum computation and astrophysics. Here, we are interested in the characterization of novel topological fermionic superfluids and possible exotic Bose-Einstein condensates (BECs) with non-trivial spin- textures. The project will investigate (i) topological (inhomogeneous) superfluids with Raman coupling; (ii) exotic superfluids in trapped BECs with linear and angular-momentum spin-orbit coupling; and (iii) Landau two-fluid hydrodynamics in spin-orbit coupled quantum gases. (2) Quantum Few-Body Systems, Fermi/Bose Polarons and Virial Expansion (Xia-Ji Liu)Quantum virial expansion is an entirely new direction to handle strong correlations, which is extremely useful at high temperatures. It allows a controllable expansion to be worked out even in three dimensions, in terms of a small parameter - the fugacity. It provides an elegant way to bridge the few-body and the many-body worlds. Our specific schemes in this topic include: (i) the four-particle solutions and molecule-molecule scattering length; (ii) the high-order virial coefficients; (iii) virial expansion study of Fermi/Bose polarons; and (iv) the high-order expansion of the single-particle spectral function and transport coefficients (i.e., shear viscosity and thermal conductivity) of a unitary Fermi gas. (3) Theory of Strongly Interacting Fermions (Hui Hu)Strongly correlated atomic gases near Feshbach resonances are notoriously difficult to understand, because of the absence of a small interaction parameter. The traditional perturbative expansion in terms of the interaction parameter is not reliable, while ab-initio Monte Carlo simulations often suffer from the Fermi sign problem. Here, we aim to (i) develop quantitative strong-coupling diagrammatic theories, (ii) perform the state-of-the-art diagrammatic quantum Monte-Carlo simulations; (iii) calculate superfluid density of unitary Fermi and Bose gases, and (iv) determine the charge-density and spin-density dynamic structure factor of a unitary Fermi gas. (4) Superfluidity in Flat Land and One Dimension (Hui Hu)Superfluidity in low-dimensional systems exhibits remarkable features, due to strong phase fluctuations. In the flat land of two dimensions, the onset of superfluidity is governed by the so-called Berezinskii-Kosterlitz-Thouless (BKT) mechanism. In one dimension, exotic inhomogeneous superfluid known as Fulde-Ferrell-Larkin-Ovchinnikov (FFLO) phase may appear. High-precision measurements in low-dimensional strongly interacting Fermi gases are now under way world-widely. In this topic, we aim to investigate (i) the thermodynamics of strongly interacting Fermi and Bose gases in flat land; (ii) the first and second sound across the BKT transition; (iii) BEC-BCS crossover in exciton-polariton condensates; and (iv) the dimensional crossover from 3D to 2D, and to 1D. (5) Grassmann phase space theory for cold Fermi gases (Bryan Dalton)This project applies phase space theory to cold Fermi gases using a non-standard version based on Grassmann phase space variables. The aim is to study phenomena such as the BEC/BCS crossover associated with Feshbach resonance, a project on which experiments are being carried out in the OSC. It is relevant to understanding the basic physics for such situations and to developing new methods for numerically treating fermion systems. Quantum correlation functions (QCF) describing the separation between fermions in a single Cooper pair and the correlation between the fermion positions in two Cooper pairs are being studied as a function of temperature for situations ranging from the BCS end of the crossover to the BEC end, with particular emphasis on the unitary regime where the fermion-fermion interactions become very strong. A theory of how to measure the QCF describing the positions in two Cooper pairs based on a four-mode version of Bragg spectroscopy is being developed. (6) Phase space theory for cold Bose gases (Bryan Dalton)This project applies standard phase space theory to cold Bose gases. The aim is to study phenomena such as discrete Floquet time crystals in periodically driven cold Bose gases, a project on which an experiment is being carried out in the OSC. It is relevant to understanding the basic physics for such systems, including how time crystals can be created. The onset of period doubling and the breakdown of period T time symmetry for a BEC dropped onto a mirror oscillating with period T is being treated using the truncated Wigner approximation in phase space theory, with the aim of studying the effects of quantum fluctuations and the breakdown of mean field theory based on the Gross-Pitaevski equation. This research is in support of the experimental project led by Professor Hannaford. |
